| | Some definitions of interest. |
|
| biject | Def Bij(A; B; f) == Inj(A; B; f) & Surj(A; B; f) |
| | | Thm*  A,B:Type, f:(A A,B:Type, f:(A  B). Bij(A; B; f) B). Bij(A; B; f)  Prop Prop |
|
| iff | Def P 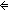  Q == (P Q == (P   Q) & (P Q) & (P 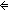  Q) Q) |
| | | Thm*  A,B:Prop. (A A,B:Prop. (A 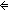  B) B)  Prop Prop |
|
| inject | Def Inj(A; B; f) ==  a1,a2:A. f(a1) = f(a2) a1,a2:A. f(a1) = f(a2)  B B   a1 = a2 a1 = a2 |
| | | Thm*  A,B:Type, f:(A A,B:Type, f:(A  B). Inj(A; B; f) B). Inj(A; B; f)  Prop Prop |
|
| is_one_one_corr | Def f is 1-1 corr ==  g:(B g:(B  A). InvFuns(A;B;f;g) A). InvFuns(A;B;f;g) |
| | | Thm*  A,B:Type, f:(A A,B:Type, f:(A  B). (f is 1-1 corr) B). (f is 1-1 corr)  Prop Prop |
|
| inv_funs_2 | Def InvFuns(A;B;f;g) == ( x:A. g(f(x)) = x) & ( x:A. g(f(x)) = x) & ( y:B. f(g(y)) = y) y:B. f(g(y)) = y) |
| | | Thm*  f:(A f:(A  B), g:(B B), g:(B  A). InvFuns(A;B;f;g) A). InvFuns(A;B;f;g)  Prop Prop |
|
| surject | Def Surj(A; B; f) ==  b:B. b:B.  a:A. f(a) = b a:A. f(a) = b |
| | | Thm*  A,B:Type, f:(A A,B:Type, f:(A  B). Surj(A; B; f) B). Surj(A; B; f)  Prop Prop |