| | Some definitions of interest. |
|
| biject | Def Bij(A; B; f) == Inj(A; B; f) & Surj(A; B; f) |
| | | Thm*  A,B:Type, f:(A A,B:Type, f:(A 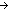 B). Bij(A; B; f) B). Bij(A; B; f) 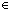 Prop Prop |
|
| double_sum | Def sum(f(x;y) | x < n; y < m) == sum(sum(f(x;y) | y < m) | x < n) |
| | | Thm*  n,m: n,m: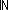 , f:( , f:(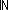 n n 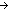 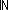 m m 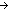 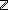 ). sum(f(x,y) | x < n; y < m) ). sum(f(x,y) | x < n; y < m) 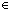 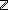 |
|
| int_seg | Def {i..j } == {k: } == {k: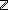 | i | i 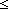 k < j } k < j } |
| | | Thm*  m,n: m,n: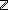 . {m..n . {m..n } } 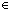 Type Type |
|
| nat | Def 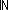 == {i: == {i: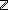 | 0 | 0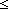 i } i } |
| | | Thm* 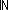 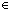 Type Type |
|
| sum | Def sum(f(x) | x < k) == primrec(k;0;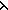 x,n. n+f(x)) x,n. n+f(x)) |
| | | Thm*  n: n: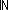 , f:( , f:(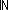 n n 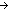 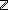 ). sum(f(x) | x < n) ). sum(f(x) | x < n) 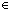 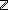 |