{  [es:EO].
[es:EO].  [P:E
[P:E 
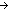
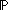 ].
].  [Q,R:E
[Q,R:E 
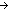 E
E 
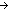
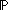 ].
].  [f:{e:E| P e}
[f:{e:E| P e} 
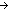 E].
E].
(Inj({e:E| P e} ;E;f)) supposing
(f is Q-R-pre-preserving on P and
AntiSym(E;e,e'.R e e') and
Refl(E;e,e'.Q e e')) }
{ Proof }
Definitions occuring in Statement :
Q-R-pre-preserving: f is Q-R-pre-preserving on P,
es-E: E,
event_ordering: EO,
anti_sym: AntiSym(T;x,y.R[x; y]),
refl: Refl(T;x,y.E[x; y]),
inject: Inj(A;B;f),
uimplies: b supposing a,
uall:  [x:A]. B[x],
prop:
[x:A]. B[x],
prop: 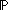 ,
set: {x:A| B[x]} ,
apply: f a,
function: x:A
,
set: {x:A| B[x]} ,
apply: f a,
function: x:A 
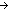 B[x]
B[x]
Definitions :
uall:  [x:A]. B[x],
prop:
[x:A]. B[x],
prop:  ,
uimplies: b supposing a,
refl: Refl(T;x,y.E[x; y]),
anti_sym: AntiSym(T;x,y.R[x; y]),
Q-R-pre-preserving: f is Q-R-pre-preserving on P,
inject: Inj(A;B;f),
all:
,
uimplies: b supposing a,
refl: Refl(T;x,y.E[x; y]),
anti_sym: AntiSym(T;x,y.R[x; y]),
Q-R-pre-preserving: f is Q-R-pre-preserving on P,
inject: Inj(A;B;f),
all:  x:A. B[x],
implies: P
x:A. B[x],
implies: P 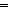
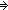 Q,
member: t
Q,
member: t  T,
and: P
T,
and: P  Q,
cand: A c
Q,
cand: A c B,
guard: {T}
B,
guard: {T}
Lemmas :
es-E_wf,
event_ordering_wf
\mforall{}[es:EO]. \mforall{}[P:E {}\mrightarrow{} \mBbbP{}]. \mforall{}[Q,R:E {}\mrightarrow{} E {}\mrightarrow{} \mBbbP{}]. \mforall{}[f:\{e:E| P e\} {}\mrightarrow{} E].
(Inj(\{e:E| P e\} ;E;f)) supposing
(f is Q-R-pre-preserving on P and
AntiSym(E;e,e'.R e e') and
Refl(E;e,e'.Q e e'))
Date html generated:
2011_08_16-AM-11_07_59
Last ObjectModification:
2011_06_18-AM-09_41_23
Home
Index