{  [A,B,S:Type].
[A,B,S:Type].  [F:S
[F:S 
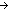 A
A 
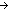 (S
(S  bag(B))].
bag(B))].  [s:S].
[s:S].  [buf:bag(B)].
[buf:bag(B)].
(seq-dataflow(rec-dataflow(s;s,a.F s
a);buffer-dataflow(buf;x.0 <z bag-size(x)))
= rec-dataflow(<s, buf>;s,a.let s1,buf = s
in let s',out = F s1 a
in <<s'
, if 0 <z bag-size(out)
then out
else buf
fi
>
, buf
>)) }
{ Proof }
Definitions occuring in Statement :
buffer-dataflow: buffer-dataflow(s;x.P[x]),
seq-dataflow: seq-dataflow(P;Q),
rec-dataflow: rec-dataflow(s0;s,m.next[s; m]),
dataflow: dataflow(A;B),
lt_int: i <z j,
ifthenelse: if b then t else f fi ,
uall:  [x:A]. B[x],
apply: f a,
function: x:A
[x:A]. B[x],
apply: f a,
function: x:A 
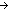 B[x],
spread: spread def,
pair: <a, b>,
product: x:A
B[x],
spread: spread def,
pair: <a, b>,
product: x:A  B[x],
natural_number: $n,
universe: Type,
equal: s = t
B[x],
natural_number: $n,
universe: Type,
equal: s = t
Lemmas :
member_wf,
top_wf,
data-stream-cons,
data-stream_wf,
squash_wf,
true_wf,
ifthenelse_wf,
dataflow_wf,
dataflow-extensionality,
seq-dataflow_wf,
rec-dataflow_wf,
buffer-dataflow_wf,
lt_int_wf,
Error :bag-size_wf,
nat_wf,
Error :bag_wf
\mforall{}[A,B,S:Type]. \mforall{}[F:S {}\mrightarrow{} A {}\mrightarrow{} (S \mtimes{} bag(B))]. \mforall{}[s:S]. \mforall{}[buf:bag(B)].
(seq-dataflow(rec-dataflow(s;s,a.F s a);buffer-dataflow(buf;x.0 <z bag-size(x)))
= rec-dataflow(<s, buf>s,a.let s1,buf = s
in let s',out = F s1 a
in <<s', if 0 <z bag-size(out) then out else buf fi >, buf>))
Date html generated:
2011_08_10-AM-08_21_16
Last ObjectModification:
2011_06_29-PM-03_19_01
Home
Index