| | Some definitions of interest. |
|
| decidable | Def Dec(P) == P 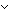  P P |
| | | Thm*  A:Prop. Dec(A) A:Prop. Dec(A)  Prop Prop |
|
| eq_int | Def i= j == if i=j j == if i=j true true ; false ; false fi fi |
| | | Thm*  i,j: i,j: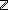 . (i= . (i= j) j)   |
|
| exists_unique | Def  !x:A. P(x) == !x:A. P(x) ==  x:A. x is the x:A. P(x) x:A. x is the x:A. P(x) |
| | | Thm*  A:Type, P:(A A:Type, P:(A  Prop). ( Prop). ( !x:A. P(x)) !x:A. P(x))  Prop Prop |
|
| int_seg | Def {i..j } == {k: } == {k: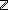 | i | i 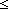 k < j } k < j } |
| | | Thm*  m,n: m,n: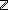 . {m..n . {m..n } }  Type Type |
|
| not | Def  A == A A == A   False False |
| | | Thm*  A:Prop. ( A:Prop. ( A) A)  Prop Prop |