Step
*
2
of Lemma
aa_bst_insert_sublemma_right
1. left_subtree : aa_ltree( )@i
)@i
2. right_subtree : aa_ltree( )@i
)@i
3. tp : aa_ltree( )@i
)@i
4. val :  @i
@i
5. i :  @i
@i
6. aa_binary_search_tree(tp)@i
7. aa_binary_search_tree(aa_lt_node(val;left_subtree;right_subtree))@i
8. i > val@i
9.  j:
j: . (aa_bst_member_prop(j;tp)
. (aa_bst_member_prop(j;tp) 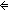

 (j = i)
(j = i)  aa_bst_member_prop(j;right_subtree))@i
aa_bst_member_prop(j;right_subtree))@i
10. j :  @i
@i
11. (j = i)  aa_bst_member_prop(j;aa_lt_node(val;left_subtree;right_subtree))@i
aa_bst_member_prop(j;aa_lt_node(val;left_subtree;right_subtree))@i
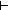 aa_bst_member_prop(j;aa_lt_node(val;left_subtree;tp))
aa_bst_member_prop(j;aa_lt_node(val;left_subtree;tp))
BY
{ InstHyp [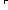 j
j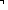 ] 9 THEN D (-1) THEN Auto' THEN BoolCase
] 9 THEN D (-1) THEN Auto' THEN BoolCase  (j =
(j = i)
i) THEN Auto'
THEN Auto' }
}
1
1. left_subtree : aa_ltree( )@i
)@i
2. right_subtree : aa_ltree( )@i
)@i
3. tp : aa_ltree( )@i
)@i
4. val :  @i
@i
5. i :  @i
@i
6. aa_binary_search_tree(tp)@i
7. aa_binary_search_tree(aa_lt_node(val;left_subtree;right_subtree))@i
8. i > val@i
9.  j:
j: . (aa_bst_member_prop(j;tp)
. (aa_bst_member_prop(j;tp) 

 (j = i)
(j = i)  aa_bst_member_prop(j;right_subtree))@i
aa_bst_member_prop(j;right_subtree))@i
10. j :  @i
@i
11. (j = i)  aa_bst_member_prop(j;aa_lt_node(val;left_subtree;right_subtree))@i
aa_bst_member_prop(j;aa_lt_node(val;left_subtree;right_subtree))@i
12. aa_bst_member_prop(j;tp) 
 ((j = i)
((j = i)  aa_bst_member_prop(j;right_subtree))
aa_bst_member_prop(j;right_subtree))
13. aa_bst_member_prop(j;tp) 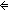
 (j = i)
(j = i)  aa_bst_member_prop(j;right_subtree)
aa_bst_member_prop(j;right_subtree)
14. j = i
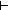 aa_bst_member_prop(j;aa_lt_node(val;left_subtree;tp))
aa_bst_member_prop(j;aa_lt_node(val;left_subtree;tp))
2
1. left_subtree : aa_ltree( )@i
)@i
2. right_subtree : aa_ltree( )@i
)@i
3. tp : aa_ltree( )@i
)@i
4. val :  @i
@i
5. i :  @i
@i
6. aa_binary_search_tree(tp)@i
7. aa_binary_search_tree(aa_lt_node(val;left_subtree;right_subtree))@i
8. i > val@i
9.  j:
j: . (aa_bst_member_prop(j;tp)
. (aa_bst_member_prop(j;tp) 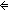

 (j = i)
(j = i)  aa_bst_member_prop(j;right_subtree))@i
aa_bst_member_prop(j;right_subtree))@i
10. j :  @i
@i
11. (j = i)  aa_bst_member_prop(j;aa_lt_node(val;left_subtree;right_subtree))@i
aa_bst_member_prop(j;aa_lt_node(val;left_subtree;right_subtree))@i
12. aa_bst_member_prop(j;tp) 
 ((j = i)
((j = i)  aa_bst_member_prop(j;right_subtree))
aa_bst_member_prop(j;right_subtree))
13. aa_bst_member_prop(j;tp) 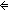
 (j = i)
(j = i)  aa_bst_member_prop(j;right_subtree)
aa_bst_member_prop(j;right_subtree)
14.  (j = i)
(j = i)
 aa_bst_member_prop(j;aa_lt_node(val;left_subtree;tp))
aa_bst_member_prop(j;aa_lt_node(val;left_subtree;tp))
1. left$_{subtree}$ : aa\_ltree(\mBbbZ{})@i
2. right$_{subtree}$ : aa\_ltree(\mBbbZ{})@i
3. tp : aa\_ltree(\mBbbZ{})@i
4. val : \mBbbZ{}@i
5. i : \mBbbZ{}@i
6. aa\_binary\_search\_tree(tp)@i
7. aa\_binary\_search\_tree(aa\_lt\_node(val;left$_{subtree}$;right$_{sub\000Ctree}$))@i
8. i > val@i
9. \mforall{}j:\mBbbZ{}. (aa\_bst\_member\_prop(j;tp) \mLeftarrow{}{}\mRightarrow{} (j = i) \mvee{} aa\_bst\_member\_prop(j;right$_{subtree\mbackslash{}ff\000C7d$))@i
10. j : \mBbbZ{}@i
11. (j = i) \mvee{} aa\_bst\_member\_prop(j;aa\_lt\_node(val;left$_{subtree}$;right$\mbackslash{}ff\000C5f{subtree}$))@i
\mvdash{} aa\_bst\_member\_prop(j;aa\_lt\_node(val;left$_{subtree}$;tp))
By
InstHyp [\mkleeneopen{}j\mkleeneclose{}] 9 THEN D (-1) THEN Auto' THEN BoolCase \mkleeneopen{}(j =\msubz{} i)\mkleeneclose{} THEN Auto'\mcdot{}
Home
Index