Step
*
2
2
2
of Lemma
not_funcNbarB_decidable_eq_fullExt
1.  t1,t2:
t1,t2:

 bar(
bar(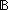 ). Dec(t1 = t2)@i
). Dec(t1 = t2)@i
2. eqd : 

 bar(
bar(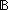 )
) 



 bar(
bar(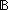 )
) 

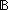
3.  t1,t2:
t1,t2:

 bar(
bar(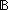 ). (
). ( (eqd t1 t2)
(eqd t1 t2) 

 t1 = t2)
t1 = t2)
4. isBot : 

 bar(
bar(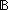 )
) 

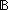
5.  t:
t:

 bar(
bar(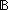 ). (
). (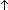 (isBot t)
(isBot t) 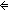

 t = (
t = ( x.bottom()))
x.bottom()))
6. isBot fix(( t,x. if isBot t then tt else bottom() fi ))
t,x. if isBot t then tt else bottom() fi )) 
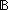
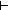 False
False
BY
{ (skip{(RW (AddrC [2;2] UnrollRecursionC) (-1) THEN Reduce (-1))}
THEN (Assert isBot fix(( t,x. if isBot t then tt else bottom() fi ))
t,x. if isBot t then tt else bottom() fi ))
= isBot ( x.if isBot fix((
x.if isBot fix(( t,x. if isBot t then tt else bottom() fi )) then tt else bottom() fi ) BY
t,x. if isBot t then tt else bottom() fi )) then tt else bottom() fi ) BY
(RW (AddrC [2;2] UnrollRecursionC) 0 THEN Reduce 0 THEN MaAuto))
) }
1
1.  t1,t2:
t1,t2:

 bar(
bar(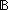 ). Dec(t1 = t2)@i
). Dec(t1 = t2)@i
2. eqd : 

 bar(
bar(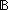 )
) 



 bar(
bar(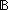 )
) 

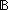
3.  t1,t2:
t1,t2:

 bar(
bar(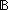 ). (
). (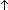 (eqd t1 t2)
(eqd t1 t2) 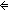

 t1 = t2)
t1 = t2)
4. isBot : 

 bar(
bar(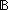 )
) 

5.  t:
t:

 bar(
bar(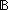 ). (
). (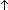 (isBot t)
(isBot t) 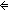

 t = (
t = ( x.bottom()))
x.bottom()))
6. isBot fix(( t,x. if isBot t then tt else bottom() fi ))
t,x. if isBot t then tt else bottom() fi )) 
7. isBot fix(( t,x. if isBot t then tt else bottom() fi ))
t,x. if isBot t then tt else bottom() fi ))
= isBot ( x.if isBot fix((
x.if isBot fix(( t,x. if isBot t then tt else bottom() fi )) then tt else bottom() fi )
t,x. if isBot t then tt else bottom() fi )) then tt else bottom() fi )
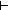 False
False
1. \mforall{}t1,t2:\mBbbN{} {}\mrightarrow{} bar(\mBbbB{}). Dec(t1 = t2)@i
2. eqd : \mBbbN{} {}\mrightarrow{} bar(\mBbbB{}) {}\mrightarrow{} \mBbbN{} {}\mrightarrow{} bar(\mBbbB{}) {}\mrightarrow{} \mBbbB{}
3. \mforall{}t1,t2:\mBbbN{} {}\mrightarrow{} bar(\mBbbB{}). (\muparrow{}(eqd t1 t2) \mLeftarrow{}{}\mRightarrow{} t1 = t2)
4. isBot : \mBbbN{} {}\mrightarrow{} bar(\mBbbB{}) {}\mrightarrow{} \mBbbB{}
5. \mforall{}t:\mBbbN{} {}\mrightarrow{} bar(\mBbbB{}). (\muparrow{}(isBot t) \mLeftarrow{}{}\mRightarrow{} t = (\mlambda{}x.bottom()))
6. isBot fix((\mlambda{}t,x. if isBot t then tt else bottom() fi )) \mmember{} \mBbbB{}
\mvdash{} False
By
(skip\{(RW (AddrC [2;2] UnrollRecursionC) (-1) THEN Reduce (-1))\}
THEN (Assert isBot fix((\mlambda{}t,x. if isBot t then tt else bottom() fi ))
= isBot
(\mlambda{}x.if isBot fix((\mlambda{}t,x. if isBot t then tt else bottom() fi ))
then tt
else bottom()
fi ) BY
(RW (AddrC [2;2] UnrollRecursionC) 0 THEN Reduce 0 THEN MaAuto))
)
Home
Index