{  es:EO
es:EO
 [T:Type]
[T:Type]
 e:E.
e:E.  f:{e':E| e'
f:{e':E| e' 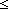 loc e }
loc e } 
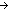 (T + Top).
(T + Top).
(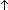 can-apply(
can-apply(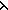 e.es-search-back(es;x.f[x];e);e)
e.es-search-back(es;x.f[x];e);e)
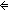
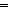
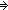
 e'
e'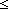 e.
e.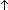 can-apply(
can-apply(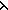 x.f[x];e')) }
x.f[x];e')) }
{ Proof }
Definitions occuring in Statement :
existse-le:  e
e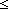 e'.P[e],
es-search-back: es-search-back(es;x.f[x];e),
es-le: e
e'.P[e],
es-search-back: es-search-back(es;x.f[x];e),
es-le: e 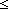 loc e' ,
es-E: E,
event_ordering: EO,
assert:
loc e' ,
es-E: E,
event_ordering: EO,
assert: 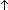 b,
uall:
b,
uall:  [x:A]. B[x],
top: Top,
so_apply: x[s],
all:
[x:A]. B[x],
top: Top,
so_apply: x[s],
all:  x:A. B[x],
iff: P
x:A. B[x],
iff: P 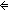
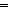
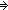 Q,
set: {x:A| B[x]} ,
lambda:
Q,
set: {x:A| B[x]} ,
lambda: 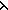 x.A[x],
function: x:A
x.A[x],
function: x:A 
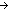 B[x],
union: left + right,
universe: Type,
can-apply: can-apply(f;x)
B[x],
union: left + right,
universe: Type,
can-apply: can-apply(f;x)
Definitions :
can-apply: can-apply(f;x),
apply: f a,
record-select: r.x,
exists:  x:A. B[x],
implies: P
x:A. B[x],
implies: P 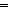
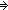 Q,
product: x:A
Q,
product: x:A  B[x],
and: P
B[x],
and: P 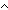 Q,
isect:
Q,
isect:  x:A. B[x],
uall:
x:A. B[x],
uall:  [x:A]. B[x],
all:
[x:A]. B[x],
all:  x:A. B[x],
iff: P
x:A. B[x],
iff: P 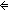
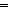
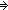 Q,
existse-le:
Q,
existse-le:  e
e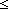 e'.P[e],
so_lambda:
e'.P[e],
so_lambda: 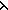
 x.t[x],
assert:
x.t[x],
assert: 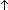 b,
union: left + right,
top: Top,
set: {x:A| B[x]} ,
es-le: e
b,
union: left + right,
top: Top,
set: {x:A| B[x]} ,
es-le: e 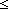 loc e' ,
es-E: E,
universe: Type,
function: x:A
loc e' ,
es-E: E,
universe: Type,
function: x:A 
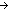 B[x],
equal: s = t,
member: t
B[x],
equal: s = t,
member: t 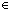 T,
event_ordering: EO,
Auto: Error :Auto,
CollapseTHENA: Error :CollapseTHENA,
CollapseTHEN: Error :CollapseTHEN,
D: Error :D,
tactic: Error :tactic,
RepeatFor: Error :RepeatFor
T,
event_ordering: EO,
Auto: Error :Auto,
CollapseTHENA: Error :CollapseTHENA,
CollapseTHEN: Error :CollapseTHEN,
D: Error :D,
tactic: Error :tactic,
RepeatFor: Error :RepeatFor
Lemmas :
event_ordering_wf,
uall_wf,
existse-le_wf,
assert_wf,
iff_wf,
top_wf,
es-le_wf,
es-E_wf,
isl-es-search-back
\mforall{}es:EO
\mforall{}[T:Type]
\mforall{}e:E. \mforall{}f:\{e':E| e' \mleq{}loc e \} {}\mrightarrow{} (T + Top).
(\muparrow{}can-apply(\mlambda{}e.es-search-back(es;x.f[x];e);e) \mLeftarrow{}{}\mRightarrow{} \mexists{}e'\mleq{}e.\muparrow{}can-apply(\mlambda{}x.f[x];e'))
Date html generated:
2011_08_16-AM-10_49_30
Last ObjectModification:
2010_11_19-PM-12_56_45
Home
Index