{ 
y:
. (
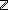w:{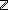| (w = ((3 * y) * (y + (3 * y))))}) }
{ Proof }
Definitions occuring in Statement :
all: x:A. B[x],
sq_exists: x:{A| B[x]},
multiply: n * m,
add: n + m,
natural_number: $n,
int: ,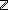
equal: s = t
Definitions :
strong-subtype: strong-subtype(A;B),
le: A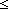 
B,
ge: i 
j ,
not: 
A,
less_than: a < b,
product: x:A 
B[x],
and: P 
Q,
uiff: uiff(P;Q),
test1,
lambda: 
x.A[x],
member: t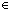 
T,
subtype_rel: A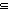 
r B,
sqequal: s ~ t,
sq_exists: x:{A| B[x]},
set: {x:A| B[x]} ,
equal: s = t,
int: ,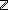
uall: [x:A]. B[x],
uimplies: b supposing a,
isect: 
x:A. B[x],
sq_type: SQType(T),
function: x:A 
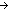B[x],
all: x:A. B[x]
Lemmas :
test1,
subtype_base_sq
\mforall{}y:\mBbbZ{}. (\mexists{}w:\{\mBbbZ{}| (w = ((3 * y) * (y + (3 * y))))\})
Date html generated:
2011_08_17-PM-05_57_25
Last ObjectModification:
2011_02_03-PM-04_27_26
Home
Index