| | Some definitions of interest. |
|
| hanoi_seq | Def s is a Hanoi(n disk) seq on a..z
Def ==  x,x':{a...z}. x,x':{a...z}.
Def == x+1 = x' 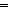 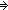 ( (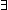 k:{1...n}. Moving disk k of n takes s(x) to s(x')) k:{1...n}. Moving disk k of n takes s(x) to s(x')) |
| | | Thm*  n: n: , a,z: , a,z: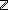 , s:({a...z} , s:({a...z} 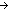 {1...n} {1...n} 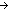 Peg). Peg).
Thm* s is a Hanoi(n disk) seq on a..z  Prop Prop |
|
| hanoi_PEG | Def Peg == {1...3} |
| | | Thm* Peg  Type Type |
|
| hanoi_seq_deepen | Def (s(?) {to n} 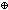 h {to n'})(x) == s(x) {to n} h {to n'})(x) == s(x) {to n} 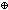 h {to n'} h {to n'} |
| | | Thm*  a,z: a,z: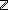 , n: , n: , s:({a...z} , s:({a...z} 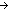 {1...n} {1...n} 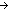 Peg), n': Peg), n': . .
Thm* n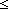 n' n'
Thm* 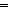 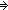
Thm* ( h:({n+1...n'} h:({n+1...n'} 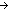 Peg). (s(?) {to n} Peg). (s(?) {to n} 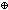 h {to n'}) h {to n'})  {a...z} {a...z} 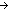 {1...n'} {1...n'} 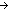 Peg) Peg) |
|
| int_iseg | Def {i...j} == {k: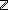 | i | i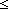 k & k k & k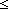 j } j } |
| | | Thm*  i,j: i,j: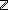 . {i...j} . {i...j}  Type Type |
|
| int_upper | Def {i...} == {j: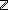 | i | i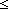 j } j } |
| | | Thm*  n: n: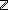 . {n...} . {n...}  Type Type |
|
| nat | Def  == {i: == {i: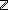 | 0 | 0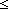 i } i } |
| | | Thm*   Type Type |
|
| le | Def A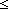 B == B ==  B<A B<A |
| | | Thm*  i,j: i,j: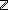 . (i . (i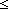 j) j)  Prop Prop |
|
| nat_plus | Def  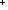 == {i: == {i: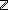 | 0<i } | 0<i } |
| | | Thm*  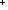  Type Type |
|
| nequal | Def a  b b  T == T ==  a = b a = b  T T |
| | | Thm*  A:Type, x,y:A. (x A:Type, x,y:A. (x  y) y)  Prop Prop |