| | Some definitions of interest. |
|
| assert | Def 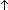 b == if b b == if b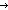 True else False fi True else False fi |
| | | Thm*  b: b: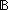 . b . b 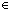 Prop Prop |
|
| is_discrete | Def A Discrete ==  x,y:A. Dec(x = y) x,y:A. Dec(x = y) |
| | | Thm*  A:Type. (A Discrete) A:Type. (A Discrete) 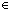 Prop Prop |
|
| decidable | Def Dec(P) == P 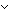 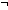 P P |
| | | Thm*  A:Prop. Dec(A) A:Prop. Dec(A) 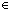 Prop Prop |
|
| iff | Def P 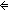  Q == (P Q == (P 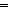  Q) & (P Q) & (P 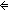 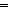 Q) Q) |
| | | Thm*  A,B:Prop. (A A,B:Prop. (A 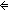  B) B) 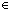 Prop Prop |