| biject |
Def Bij(A; B; f) == Inj(A; B; f) & Surj(A; B; f)
Thm*  A,B:Type, f:(A A,B:Type, f:(A 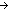 B). Bij(A; B; f) B). Bij(A; B; f) 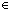 Prop Prop
|
| exp |
Def (base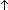 power) == if power= power) == if power=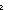 0 0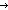 1 else base 1 else base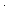 (base (base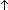 power-1) fi (recursive) power-1) fi (recursive)
Thm*  n,k: n,k: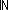 . (n . (n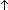 k) k) 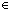 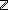
Thm*  n,k: n,k: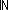 . (n . (n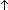 k) k) 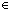 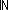
|
| int_seg |
Def {i..j } == {k: } == {k: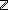 | i | i 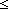 k < j } k < j }
Thm*  m,n: m,n: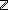 . {m..n . {m..n } } 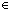 Type Type
|
| length |
Def ||as|| == Case of as; nil 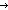 0 ; a.as' 0 ; a.as' 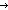 ||as'||+1 (recursive) ||as'||+1 (recursive)
Thm*  A:Type, l:A*. ||l|| A:Type, l:A*. ||l|| 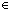 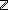
Thm* ||nil|| 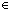 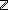
|
| nat |
Def 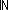 == {i: == {i: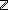 | 0 | 0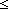 i } i }
Thm* 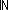 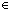 Type Type
|
| surject |
Def Surj(A; B; f) ==  b:B. b:B. 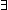 a:A. f(a) = b a:A. f(a) = b
Thm*  A,B:Type, f:(A A,B:Type, f:(A 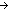 B). Surj(A; B; f) B). Surj(A; B; f) 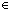 Prop Prop
|
| inject |
Def Inj(A; B; f) ==  a1,a2:A. f(a1) = f(a2) a1,a2:A. f(a1) = f(a2) 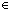 B B 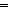 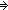 a1 = a2 a1 = a2
Thm*  A,B:Type, f:(A A,B:Type, f:(A 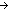 B). Inj(A; B; f) B). Inj(A; B; f) 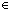 Prop Prop
|
| eq_int |
Def i=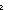 j == if i=j j == if i=j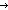 true true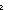 ; false ; false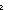 fi fi
Thm*  i,j: i,j: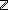 . i= . i=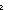 j j 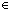 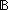
|
| lelt |
Def i 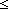 j < k == i j < k == i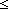 j & j < k j & j < k
|
| le |
Def A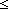 B == B == 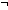 B < A B < A
Thm*  i,j: i,j: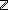 . i . i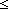 j j 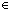 Prop Prop
|
| not |
Def 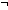 A == A A == A 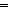 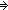 False False
Thm*  A:Prop. ( A:Prop. (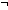 A) A) 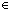 Prop Prop
|
![]() A,B:Type, f:(A
A,B:Type, f:(A![]()
![]() B). Bij(A; B; f)
B). Bij(A; B; f) ![]() Prop
Prop![]() n,k:
n,k:![]() . (n
. (n![]() k)
k) ![]()
![]()
![]() n,k:
n,k:![]() . (n
. (n![]() k)
k) ![]()
![]()
![]() m,n:
m,n:![]() . {m..n
. {m..n![]() }
} ![]() Type
Type![]() A:Type, l:A*. ||l||
A:Type, l:A*. ||l|| ![]()
![]()
![]()
![]()
![]()
![]() Type
Type![]() A,B:Type, f:(A
A,B:Type, f:(A![]()
![]() B). Surj(A; B; f)
B). Surj(A; B; f) ![]() Prop
Prop![]() A,B:Type, f:(A
A,B:Type, f:(A![]()
![]() B). Inj(A; B; f)
B). Inj(A; B; f) ![]() Prop
Prop![]() i,j:
i,j:![]() . i=
. i=![]() j
j ![]()
![]()
![]() i,j:
i,j:![]() . i
. i![]() j
j ![]() Prop
Prop![]() A:Prop. (
A:Prop. (![]() A)
A) ![]() Prop
Prop