| | Some definitions of interest. |
|
| assert | Def 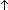 b == if b b == if b True else False fi True else False fi |
| | | Thm*  b: b: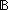 . b . b 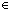 Prop Prop |
|
| search | Def search(k;P) == primrec(k;0;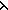 i,j. if 0< i,j. if 0<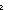 j j j ; P(i) j ; P(i) i+1 else 0 fi) i+1 else 0 fi) |
| | | Thm*  k: k: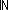 , P:( , P:(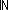 k k 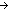 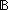 ). search(k;P) ). search(k;P) 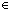 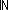 (k+1) (k+1) |
|
| swap | Def swap(L;i;j) == (L o (i, j)) |
| | | Thm*  T:Type, L:T List, i,j: T:Type, L:T List, i,j: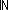 ||L||. swap(L;i;j) ||L||. swap(L;i;j) 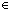 T List T List |
|
| eq_int | Def i=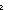 j == if i=j j == if i=j true true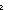 ; false ; false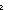 fi fi |
| | | Thm*  i,j: i,j: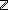 . (i= . (i=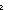 j) j) 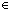 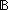 |
|
| int_seg | Def {i..j } == {k: } == {k: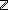 | i | i 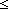 k < j } k < j } |
| | | Thm*  m,n: m,n: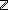 . {m..n . {m..n } } 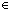 Type Type |
|
| nat | Def 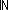 == {i: == {i: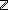 | 0 | 0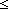 i } i } |
| | | Thm* 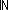 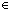 Type Type |
|
| le | Def A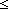 B == B ==  B<A B<A |
| | | Thm*  i,j: i,j: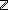 . (i . (i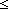 j) j) 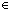 Prop Prop |
|
| length | Def ||as|| == Case of as; nil  0 ; a.as' 0 ; a.as'  ||as'||+1 (recursive) ||as'||+1 (recursive) |
| | | Thm*  A:Type, l:A List. ||l|| A:Type, l:A List. ||l|| 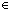 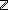 |
| | | Thm* ||nil|| 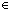 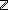 |
|
| let | Def let x = a in b(x) == (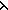 x.b(x))(a) x.b(x))(a) |
| | | Thm*  A,B:Type, a:A, b:(A A,B:Type, a:A, b:(A 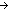 B). let x = a in b(x) B). let x = a in b(x) 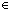 B B |
|
| not | Def  A == A A == A 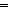 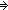 False False |
| | | Thm*  A:Prop. ( A:Prop. ( A) A) 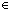 Prop Prop |
|
| null | Def null(as) == Case of as; nil  true true ; a.as' ; a.as'  false false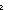 |
| | | Thm*  T:Type, as:T List. null(as) T:Type, as:T List. null(as) 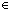 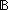 |
| | | Thm* null(nil) 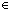  |