At: M DASH C DASH S SPACE induction 2 2 1 2 1 2 1 1 1
1. E: TaggedEventStruct
2. P: (|E| List)
 Prop
Prop
3. I: (|E| List)
 Prop
Prop
4. memorylessR(E) preserves P
5.  x,y,z:|E| List. P(x)
x,y,z:|E| List. P(x) 
 P(y)
P(y) 
 (
( x
x x.(
x.( y
y y.
y. (x =msg=(E) y))) & z = (x @ y)
(x =msg=(E) y))) & z = (x @ y) 
 P(z)
P(z)
6.  x,y:|E| List. P(x)
x,y:|E| List. P(x) 
 y
y 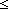 x
x 
 P(y)
P(y)
7. safetyR(E) preserves I
8.  tr:|E| List.
I(tr)
tr:|E| List.
I(tr) 

 tr = nil
tr = nil 
 (
(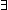 L_1,L_2:|E| List.
tr = (L_1 @ L_2)
&
L_1,L_2:|E| List.
tr = (L_1 @ L_2)
&  L_2 = nil
& (
L_2 = nil
& ( x
x L_1.(
L_1.( y
y L_2.
L_2. (x =msg=(E) y)))
& (
(x =msg=(E) y)))
& (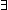 m:Label. (
m:Label. ( x
x L_2.tag(E)(x) = m)))
L_2.tag(E)(x) = m)))
9. n: 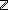
10. 0 < n
11.  tr:|E| List. ||tr||
tr:|E| List. ||tr||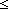 n-1
n-1 
 (
( m:Label. P( < tr > _m))
m:Label. P( < tr > _m)) 
 I(tr)
I(tr) 
 P(tr)
P(tr)
12. tr: |E| List
13. ||tr||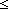 n
n
14.  tr = nil
tr = nil
15.  m:Label. P( < tr > _m)
m:Label. P( < tr > _m)
16. I(tr)
17. L_1: |E| List
18. L_2: |E| List
19. tr = (L_1 @ L_2)
20.  L_2 = nil
L_2 = nil
21. ( x
x L_1.(
L_1.( y
y L_2.
L_2. (x =msg=(E) y)))
(x =msg=(E) y)))
22. m: Label
23. ( x
x L_2.tag(E)(x) = m)
L_2.tag(E)(x) = m)
24. ||L_1||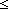 n-1
n-1
25. L_1 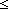 tr
tr
26. I(L_1)
27. ||L_1||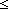 n-1
n-1
28. L_1 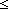 tr
tr
29. I(L_1)
30. ( m:Label. P( < L_1 > _m))
m:Label. P( < L_1 > _m)) 
 I(L_1)
I(L_1) 
 P(L_1)
P(L_1)
31. m1: Label
32. P( < tr > _m1)
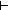 < L_1 > _m1
< L_1 > _m1 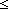 < tr > _m1
< tr > _m1
By:
Unfold `tag_sublist` 0
THEN
BackThru
Thm*  P:(T
P:(T

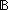 ), L2,L1:T List. L1
), L2,L1:T List. L1 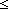 L2
L2 
 filter(P;L1)
filter(P;L1) 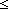 filter(P;L2)
filter(P;L2)
Generated subgoals:None
About: