| | Who Cites single tag decomposable? |
|
| single_tag_decomposable | Def single-tag-decomposable(E)(L)
==  L = nil L = nil  |E| List |E| List   (
(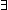 L_1,L_2:Trace(E).
L = (L_1 @ L_2) L_1,L_2:Trace(E).
L = (L_1 @ L_2)  |E| List
& |E| List
&  L_2 = nil L_2 = nil  |E| List
& ( |E| List
& ( x x L_1.( L_1.( y y L_2. L_2.  (x =msg=(E) y)))
& ( (x =msg=(E) y)))
& (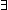 m:Label. ( m:Label. ( x x L_2.tag(E)(x) = m))) L_2.tag(E)(x) = m))) |
|
| event_tag |
Def tag(E) == 1of(2of(2of(2of(2of(2of(E)))))) |
| | |
Thm*  E:TaggedEventStruct. tag(E) E:TaggedEventStruct. tag(E)  |E| |E|  Label Label |
|
| lbl |
Def Label == {p:Pattern|  ground_ptn(p) } ground_ptn(p) } |
| | | Thm* Label  Type Type |
|
| str_trace |
Def Trace(E) == |E| List |
|
| carrier |
Def |S| == 1of(S) |
| | | Thm*  S:Structure. |S| S:Structure. |S|  Type Type |
|
| l_all |
Def ( x x L.P(x)) == L.P(x)) ==  x:T. (x x:T. (x  L) L)   P(x) P(x) |
| | | Thm*  T:Type, L:T List, P:(T T:Type, L:T List, P:(T  Prop). ( Prop). ( x x L.P(x)) L.P(x))  Prop Prop |
|
| event_msg_eq |
Def =msg=(E)(e_1,e_2) == (msg(E)(e_1)) =(MS(E)) (msg(E)(e_2)) |
| | | Thm*  E:EventStruct. =msg=(E) E:EventStruct. =msg=(E)  |E| |E|  |E| |E|  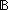 |
|
| assert |
Def  b == if b b == if b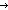 True else False fi True else False fi |
| | | Thm*  b: b: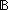 . b . b  Prop Prop |
|
| l_member |
Def (x  l) == l) == 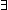 i: i: . i < ||l|| & x = l[i] . i < ||l|| & x = l[i]  T T |
| | | Thm*  T:Type, x:T, l:T List. (x T:Type, x:T, l:T List. (x  l) l)  Prop Prop |
|
| nat |
Def  == {i: == {i: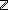 | 0 | 0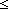 i } i } |
| | | Thm*   Type Type |
|
| le |
Def A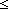 B == B ==  B < A B < A |
| | | Thm*  i,j: i,j: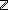 . (i . (i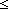 j) j)  Prop Prop |
|
| not |
Def  A == A A == A   False False |
| | | Thm*  A:Prop. ( A:Prop. ( A) A)  Prop Prop |
|
| append |
Def as @ bs == Case of as; nil 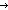 bs ; a.as' bs ; a.as' 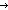 [a / (as' @ bs)] (recursive) [a / (as' @ bs)] (recursive) |
| | |
Thm*  T:Type, as,bs:T List. (as @ bs) T:Type, as,bs:T List. (as @ bs)  T List T List |
|
| event_msg |
Def msg(E) == 1of(2of(2of(E))) |
| | |
Thm*  E:EventStruct. msg(E) E:EventStruct. msg(E)  |E| |E|  |MS(E)| |MS(E)| |
|
| event_msg_str |
Def MS(E) == 1of(2of(E)) |
| | | Thm*  E:EventStruct. MS(E) E:EventStruct. MS(E)  MessageStruct MessageStruct |
|
| msg_eq |
Def =(M)(m_1,m_2)
== ((content(M)(m_1)) =(cEQ(M)) (content(M)(m_2)))  sender(M)(m_1) = sender(M)(m_1) = sender(M)(m_2) sender(M)(m_2)  (uid(M)(m_1)=
(uid(M)(m_1)= uid(M)(m_2)) uid(M)(m_2)) |
| | | Thm*  M:MessageStruct. =(M) M:MessageStruct. =(M)  |M| |M|  |M| |M|  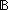 |
|
| msg_id |
Def uid(MS) == 1of(2of(2of(2of(2of(MS))))) |
| | |
Thm*  M:MessageStruct. uid(M) M:MessageStruct. uid(M)  |M| |M|  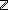 |
|
| msg_sender |
Def sender(MS) == 1of(2of(2of(2of(MS)))) |
| | |
Thm*  M:MessageStruct. sender(M) M:MessageStruct. sender(M)  |M| |M|  Label Label |
|
| msg_content |
Def content(MS) == 1of(2of(2of(MS))) |
| | |
Thm*  M:MessageStruct. content(M) M:MessageStruct. content(M)  |M| |M|  |cEQ(M)| |cEQ(M)| |
|
| msg_content_eq |
Def cEQ(MS) == 1of(2of(MS)) |
| | | Thm*  M:MessageStruct. cEQ(M) M:MessageStruct. cEQ(M)  DecidableEquiv DecidableEquiv |
|
| eq_dequiv |
Def =(DE) == 1of(2of(DE)) |
| | | Thm*  E:DecidableEquiv. =(E) E:DecidableEquiv. =(E)  |E| |E|  |E| |E|  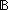 |
|
| pi2 |
Def 2of(t) == t.2 |
| | |
Thm*  A:Type, B:(A A:Type, B:(A  Type), p:(a:A Type), p:(a:A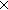 B(a)). 2of(p) B(a)). 2of(p)  B(1of(p)) B(1of(p)) |
|
| pi1 |
Def 1of(t) == t.1 |
| | | Thm*  A:Type, B:(A A:Type, B:(A  Type), p:(a:A Type), p:(a:A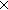 B(a)). 1of(p) B(a)). 1of(p)  A A |
|
| ground_ptn |
Def ground_ptn(p)
== Case(p)
Case ptn_var(v) = >
false Case ptn_pr( < x, y > ) = >
ground_ptn(x)
Case ptn_pr( < x, y > ) = >
ground_ptn(x)  ground_ptn(y)
Default = > true ground_ptn(y)
Default = > true (recursive)
(recursive) |
| | |
Thm*  p:Pattern. ground_ptn(p) p:Pattern. ground_ptn(p)  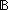 |
|
| ptn |
Def Pattern == rec(T.ptn_con(T)) |
| | |
Thm* Pattern  Type Type |
|
| eq_lbl |
Def l1 = l2
== Case(l1)
Case ptn_atom(x) = >
Case(l2)
Case ptn_atom(y) = >
x= l2
== Case(l1)
Case ptn_atom(x) = >
Case(l2)
Case ptn_atom(y) = >
x= y y Atom
Default = > false Atom
Default = > false Case ptn_int(x) = >
Case(l2)
Case ptn_int(y) = >
x=
Case ptn_int(x) = >
Case(l2)
Case ptn_int(y) = >
x= y
Default = > false y
Default = > false Case ptn_var(x) = >
Case(l2)
Case ptn_var(y) = >
x=
Case ptn_var(x) = >
Case(l2)
Case ptn_var(y) = >
x= y y Atom
Default = > false Atom
Default = > false Case ptn_pr( < x, y > ) = >
Case(l2)
Case ptn_pr( < u, v > ) = >
x =
Case ptn_pr( < x, y > ) = >
Case(l2)
Case ptn_pr( < u, v > ) = >
x = u u  y = y = v
Default = > false v
Default = > false Default = > false
Default = > false (recursive)
(recursive) |
| | |
Thm*  l1,l2:Pattern. l1 = l1,l2:Pattern. l1 = l2 l2  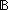 |
|
| case_default |
Def Default = > body(value,value) == body |
|
| band |
Def p  q == if p q == if p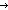 q else false q else false fi fi |
| | | Thm*  p,q: p,q: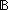 . (p . (p  q) q)  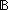 |
|
| case_lbl_pair |
Def Case ptn_pr( < x, y > ) = > body(x;y) cont(x1,z)
== InjCase(x1; _. cont(z,z); x2.
InjCase(x2; _. cont(z,z); x2@0. InjCase(x2@0; _. cont(z,z); x2@1. x2@1/x3,x2@2. body(x3;x2@2)))) |
|
| case_ptn_var |
Def Case ptn_var(x) = > body(x) cont(x1,z)
== (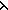 x1.inr(x2) = >
( x1.inr(x2) = >
(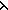 x1.inr(x2) = >
( x1.inr(x2) = >
(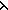 x1.inl(x2) = > body(hd([x2 / tl(x1)])) cont(hd(x1),z))([x2 / tl(x1)])
cont
(hd(x1)
,z))
([x2 / tl(x1)])
cont
(hd(x1)
,z))
([x1]) x1.inl(x2) = > body(hd([x2 / tl(x1)])) cont(hd(x1),z))([x2 / tl(x1)])
cont
(hd(x1)
,z))
([x2 / tl(x1)])
cont
(hd(x1)
,z))
([x1]) |
|
| case |
Def Case(value) body == body(value,value) |
|
| ptn_con |
Def ptn_con(T) == Atom+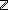 +Atom+(T +Atom+(T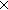 T) T) |
| | | Thm*  T:Type. ptn_con(T) T:Type. ptn_con(T)  Type Type |
|
| select |
Def l[i] == hd(nth_tl(i;l)) |
| | |
Thm*  A:Type, l:A List, n: A:Type, l:A List, n: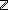 . 0 . 0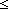 n n   n < ||l|| n < ||l||   l[n] l[n]  A A |
|
| length |
Def ||as|| == Case of as; nil 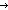 0 ; a.as' 0 ; a.as' 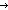 ||as'||+1 (recursive) ||as'||+1 (recursive) |
| | |
Thm*  A:Type, l:A List. ||l|| A:Type, l:A List. ||l||  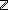 |
| | |
Thm* ||nil||  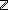 |
|
| eq_int |
Def i= j == if i=j j == if i=j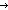 true true ; false ; false fi fi |
| | | Thm*  i,j: i,j: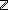 . (i= . (i= j) j)  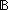 |
|
| case_ptn_int |
Def Case ptn_int(x) = > body(x) cont(x1,z)
== (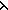 x1.inr(x2) = >
( x1.inr(x2) = >
(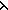 x1.inl(x2) = > body(hd([x2 / tl(x1)])) cont(hd(x1),z))([x2 / tl(x1)])
cont
(hd(x1)
,z))
([x1]) x1.inl(x2) = > body(hd([x2 / tl(x1)])) cont(hd(x1),z))([x2 / tl(x1)])
cont
(hd(x1)
,z))
([x1]) |
|
| hd |
Def hd(l) == Case of l; nil 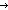 "?" ; h.t "?" ; h.t 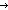 h h |
| | |
Thm*  A:Type, l:A List. ||l|| A:Type, l:A List. ||l|| 1 1   hd(l) hd(l)  A A |
| | |
Thm*  A:Type, l:A List A:Type, l:A List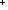 . hd(l) . hd(l)  A A |
|
| nth_tl |
Def nth_tl(n;as) == if n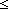  0 0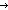 as else nth_tl(n-1;tl(as)) fi (recursive) as else nth_tl(n-1;tl(as)) fi (recursive) |
| | |
Thm*  A:Type, as:A List, i: A:Type, as:A List, i: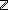 . nth_tl(i;as) . nth_tl(i;as)  A List A List |
|
| tl |
Def tl(l) == Case of l; nil 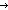 nil ; h.t nil ; h.t 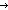 t t |
| | |
Thm*  A:Type, l:A List. tl(l) A:Type, l:A List. tl(l)  A List A List |
|
| case_inl |
Def inl(x) = > body(x) cont(value,contvalue)
== InjCase(value; x. body(x); _. cont(contvalue,contvalue)) |
|
| case_inr |
Def inr(x) = > body(x) cont(value,contvalue)
== InjCase(value; _. cont(contvalue,contvalue); x. body(x)) |
|
| eq_atom |
Def x= y y Atom == if x=y Atom == if x=y Atom Atom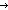 true true ; false ; false fi fi |
| | | Thm*  x,y:Atom. x= x,y:Atom. x= y y Atom Atom  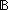 |
|
| case_ptn_atom |
Def Case ptn_atom(x) = > body(x) cont(x1,z)
== InjCase(x1; x2. body(x2); _. cont(z,z)) |
|
| le_int |
Def i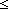  j == j ==   j < j <  i i |
| | | Thm*  i,j: i,j: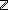 . (i . (i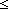  j) j)  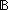 |
|
| lt_int |
Def i <  j == if i < j j == if i < j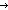 true true ; false ; false fi fi |
| | | Thm*  i,j: i,j: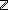 . (i < . (i <  j) j)  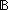 |
|
| bnot |
Def   b == if b b == if b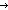 false false else true else true fi fi |
| | | Thm*  b: b: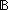 . .   b b  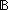 |