Thm*  E:EventStruct, tr:|E| List, ls: E:EventStruct, tr:|E| List, ls: ||tr||.
is-send(E)(tr[ls]) ||tr||.
is-send(E)(tr[ls])   (
( j: j: ||tr||. ls < j ||tr||. ls < j    is-send(E)(tr[j])) is-send(E)(tr[j]))   (
( i,j: i,j: ||tr||. i ||tr||. i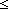 j j   is-send(E)(tr[j]) is-send(E)(tr[j])   (i (switchR(tr)^*) ls) (i (switchR(tr)^*) ls)   (j (switchR(tr)^*) ls)) (j (switchR(tr)^*) ls)) | [switch_inv_rel_closure_lemma1] |
Thm*  E:EventStruct, P:((Label E:EventStruct, P:((Label  (|E| List)) (|E| List))  Prop).
( Prop).
( f,g:(Label f,g:(Label  (|E| List)). ( (|E| List)). ( p:Label. g(p) p:Label. g(p) 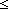 f(p)) f(p))   P(f) P(f)   P(g)) P(g))   (
( f,g:(Label f,g:(Label  (|E| List)).
( (|E| List)).
(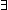 a:|E|. a:|E|.  p:Label. g(p) = filter( p:Label. g(p) = filter(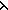 b. b.  (b =msg=(E) a);f(p))) (b =msg=(E) a);f(p)))   P(f) P(f)   P(g)) P(g))
  (
( f,g,h:(Label f,g,h:(Label  (|E| List)).
( (|E| List)).
( p,q:Label. ( p,q:Label. ( x x f(p).( f(p).( y y g(q). g(q). (x =msg=(E) y)))) (x =msg=(E) y))))   (
( p:Label. h(p) = ((f(p)) @ (g(p)))) p:Label. h(p) = ((f(p)) @ (g(p))))   P(f) P(f)   P(g) P(g)   P(h)) P(h))
  switchable0(E)(local_deliver_property(E;P))
switchable0(E)(local_deliver_property(E;P)) | [local_deliver_switchable] |
Thm*  E:TaggedEventStruct, x:|E| List, i: E:TaggedEventStruct, x:|E| List, i: (||x||-1).
switch_inv(E)(x) (||x||-1).
switch_inv(E)(x)  
 is-send(E)(x[(i+1)]) is-send(E)(x[(i+1)])   is-send(E)(x[i])
is-send(E)(x[i]) 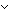  loc(E)(x[i]) = loc(E)(x[(i+1)]) loc(E)(x[i]) = loc(E)(x[(i+1)])   switch_inv(E)(swap(x;i;i+1)) switch_inv(E)(swap(x;i;i+1)) | [switch_inv_swap] |
Thm*  E:EventStruct, a,b:|E|, tr:|E| List. E:EventStruct, a,b:|E|, tr:|E| List.
 a somewhere delivered before b a somewhere delivered before b
  (
( k: k: ||tr||.
a delivered at time k ||tr||.
a delivered at time k   (
(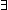 k': k': ||tr||. k' < k & b delivered at time k' & loc(E)(tr[k']) = loc(E)(tr[k]))) ||tr||. k' < k & b delivered at time k' & loc(E)(tr[k']) = loc(E)(tr[k]))) | [not_delivered_before_somewhere] |
Thm*  E:EventStruct, L:|E| List. E:EventStruct, L:|E| List.
 L = nil L = nil   Causal(E)(L) Causal(E)(L)   ( (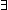 i: i: ||L||. is-send(E)(L[i])) ||L||. is-send(E)(L[i])) | [P_causal_non_nil] |
Thm*  E:EventStruct, tr:|E| List.
No-dup-deliver(E)(tr) E:EventStruct, tr:|E| List.
No-dup-deliver(E)(tr)
  (
( x,y:|E|. x,y:|E|.
 is-send(E)(x) is-send(E)(x)  
 is-send(E)(y) is-send(E)(y)   (y =msg=(E) x) (y =msg=(E) x)   loc(E)(x) = loc(E)(y) loc(E)(x) = loc(E)(y)    sublist(|E|;[x; y];tr)) sublist(|E|;[x; y];tr)) | [P_no_dup_iff] |
Thm*  msg:(A msg:(A  A A  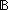 ), L1,L2:A List.
( ), L1,L2:A List.
( a,b:A. (a a,b:A. (a  L1) L1)   (b (b  L2) L2)    msg(a,b)) msg(a,b))   (L1 -msg(a,b) L2) = L1 (L1 -msg(a,b) L2) = L1 | [remove_msgs_disjoint] |
Def switch_inv(E)(tr)
==  i,j,k: i,j,k: ||tr||.
i < j ||tr||.
i < j  
 (is-send(E)(tr[i])) (is-send(E)(tr[i]))  
 (is-send(E)(tr[j])) (is-send(E)(tr[j]))  
 tag(E)(tr[i]) = tag(E)(tr[j]) tag(E)(tr[i]) = tag(E)(tr[j])   tr[j] delivered at time k
tr[j] delivered at time k   (
(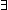 k': k': ||tr||. k' < k & tr[i] delivered at time k' & loc(E)(tr[k']) = loc(E)(tr[k])) ||tr||. k' < k & tr[i] delivered at time k' & loc(E)(tr[k']) = loc(E)(tr[k])) | [switch_inv] |
Def asyncR(E)
== swap adjacent[ loc(E)(x) = loc(E)(y)
& loc(E)(x) = loc(E)(y)
&   (is-send(E)(x)) & (is-send(E)(x)) &   (is-send(E)(y)) (is-send(E)(y)) 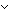  (is-send(E)(x)) & (is-send(E)(x)) &  (is-send(E)(y))] (is-send(E)(y))] | [R_async] |
Def delayableR(E)
== swap adjacent[  (x =msg=(E) y)
& (x =msg=(E) y)
&   (is-send(E)(x)) & (is-send(E)(x)) &  (is-send(E)(y)) (is-send(E)(y)) 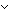  (is-send(E)(x)) & (is-send(E)(x)) &   (is-send(E)(y))] (is-send(E)(y))] | [R_delayable] |
Def AD-normal(E)(tr)
==  i: i: (||tr||-1).
( (||tr||-1).
( (is-send(E)(tr[i])) (is-send(E)(tr[i]))     (is-send(E)(tr[(i+1)])) (is-send(E)(tr[(i+1)]))    (tr[i] =msg=(E) tr[(i+1)]))
& (( (tr[i] =msg=(E) tr[(i+1)]))
& ((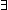 x,y: x,y: ||tr||.
x < y
& ||tr||.
x < y
&  (is-send(E)(tr[x]))
& (is-send(E)(tr[x]))
&  (is-send(E)(tr[y]))
& tr[x] delivered at time i+1
& tr[y] delivered at time i) (is-send(E)(tr[y]))
& tr[x] delivered at time i+1
& tr[y] delivered at time i)
  loc(E)(tr[i]) = loc(E)(tr[(i+1)]))
loc(E)(tr[i]) = loc(E)(tr[(i+1)])) | [switch_normal] |
Def R(tg) == swap adjacent[ tg(x) = tg(y) tg(x) = tg(y)  Label]^* Label]^* | [tag_rel] |
Def Macro
x R_del(E) y
==   (x =msg=(E) y)
& is-deliver(E)(x) & (x =msg=(E) y)
& is-deliver(E)(x) &  (is-send(E)(y)) (is-send(E)(y)) 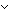  (is-send(E)(x)) & is-deliver(E)(y) (is-send(E)(x)) & is-deliver(E)(y) | [R_del] |
Def composableR(E)(L_1,L_2,L)
== ( x x L_1.( L_1.( y y L_2. L_2.  (x =msg=(E) y))) & L = (L_1 @ L_2) (x =msg=(E) y))) & L = (L_1 @ L_2)  |E| List |E| List | [R_composable] |
Def R_ad_normal(tr)(a,b)
== ( (is-send(E)(a)) (is-send(E)(a))     (is-send(E)(b)) (is-send(E)(b))    (a =msg=(E) b))
& ( (a =msg=(E) b))
& (  (is-send(E)(a)) (is-send(E)(a))  
  (is-send(E)(b)) (is-send(E)(b))   (
(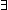 x,y: x,y: ||tr||.
x < y
& ||tr||.
x < y
&  (is-send(E)(tr[x]))
& (is-send(E)(tr[x]))
&  (is-send(E)(tr[y]))
& (is-send(E)(tr[y]))
&  (tr[x] =msg=(E) b)
& (tr[x] =msg=(E) b)
&  (tr[y] =msg=(E) a)) (tr[y] =msg=(E) a))
  loc(E)(a) = loc(E)(b))
loc(E)(a) = loc(E)(b)) | [R_ad_normal] |
Def single-tag-decomposable(E)(L)
==  L = nil L = nil  |E| List |E| List   (
(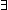 L_1,L_2:Trace(E).
L = (L_1 @ L_2) L_1,L_2:Trace(E).
L = (L_1 @ L_2)  |E| List
& |E| List
&  L_2 = nil L_2 = nil  |E| List
& ( |E| List
& ( x x L_1.( L_1.( y y L_2. L_2.  (x =msg=(E) y)))
& ( (x =msg=(E) y)))
& (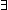 m:Label. ( m:Label. ( x x L_2.tag(E)(x) = m))) L_2.tag(E)(x) = m))) | [single_tag_decomposable] |
Def No-dup-deliver(E)(tr)
==  i,j: i,j: ||tr||. ||tr||.
  (is-send(E)(tr[i])) (is-send(E)(tr[i]))  
  (is-send(E)(tr[j])) (is-send(E)(tr[j]))    (tr[j] =msg=(E) tr[i]) (tr[j] =msg=(E) tr[i])   loc(E)(tr[i]) = loc(E)(tr[j]) loc(E)(tr[i]) = loc(E)(tr[j])   i = j i = j | [P_no_dup] |
Def x delivered at time k ==  (x =msg=(E) tr[k]) & (x =msg=(E) tr[k]) &   (is-send(E)(tr[k])) (is-send(E)(tr[k])) | [delivered_at] |
Def switch_inv(E; tr)
==  i,j,k: i,j,k: ||tr||.
i < j ||tr||.
i < j  
 (is-send(E)(tr[i])) (is-send(E)(tr[i]))  
 (is-send(E)(tr[j])) (is-send(E)(tr[j]))  
 tag(E)(tr[i]) = tag(E)(tr[j]) tag(E)(tr[i]) = tag(E)(tr[j])  
 (tr[j] =msg=(E) tr[k]) (tr[j] =msg=(E) tr[k])  
  (is-send(E)(tr[k])) (is-send(E)(tr[k]))   (
(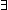 k': k': ||tr||.
k' < k & loc(E)(tr[k']) = loc(E)(tr[k]) & ||tr||.
k' < k & loc(E)(tr[k']) = loc(E)(tr[k]) &  (tr[i] =msg=(E) tr[k']) & (tr[i] =msg=(E) tr[k']) &   (is-send(E)(tr[k']))) (is-send(E)(tr[k']))) | [switch_inv2001_03_15_DASH_PM_DASH_12_53_21] |